User:
Lift coefficient
Description
The lift coefficient is a function of the angle of attack and typically follows the trend indicated in the following figure:
In the illustrated case, the slope is approximately 1.5 per 15 degrees, which corresponds to 0.1 1/degree or 5.73 1/radian.
ID:(7148, 0)
Variation of the lift coefficient
Description
Both airplanes and birds can modify the shape of their wings. Airplanes achieve this through the use of flaps, while birds adjust the position of their primary and secondary feathers. This allows them to achieve high lift at low speeds during takeoff and landing, and a reduced lift coefficient at high speeds.
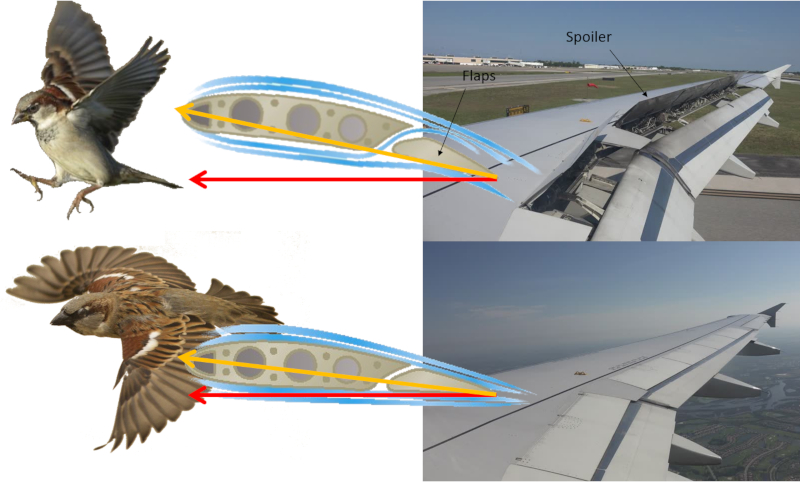
Additionally, airplanes are equipped with spoilers that assist in braking during landing.
ID:(11072, 0)
Flight condition
Equation
In order for a spacecraft or a bird to remain in flight, the gravitational force ($F_g$) must counteract the force of gravity, which is defined by the mass of Object ($m$) and the gravitational Acceleration ($g$). In other words, it must be:
 $ F_g = m g $ |
This is a simplified situation that does not take into account that the force of resistance can also generate a lift force.
ID:(14515, 0)
Lift force
Equation
To generate higher pressure below than above the wing and generate lift, Bernoulli's principle is employed, correcting for the lack of energy density conservation using ($$). The pressure over the wing, the lift force ($F_L$), can be estimated using the density ($\rho$), the surface that generates lift ($S_w$), the coefficient of lift ($C_L$), and the speed with respect to the medium ($v$) through the following formula:
| $ F_L =\displaystyle\frac{1}{2} \rho S_w C_L v ^2$ |
The lift force ($F_L$), along with the wing span ($L$), the density ($\rho$), the wing top speed factor ($c_t$), the wing bottom speed factor ($c_b$), the upper wing length ($l_t$), the bottom wing length ($l_b$), and the speed with respect to the medium ($v$), is found in
| $ F_L = \rho L ( c_b l_b - c_t l_t ) v ^2$ |
If we consider the surface that generates lift ($S_w$), given by the wing span ($L$), the upper wing length ($l_t$), and the bottom wing length ($l_b$),
| $ S_w = \displaystyle\frac{1}{2} L ( l_t + l_b )$ |
and for the coefficient of lift ($C_L$), defined as
| $ C_L = 4\displaystyle\frac{ c_t l_t - c_b l_b }{ l_t + l_b }$ |
we obtain
| $ F_L =\displaystyle\frac{1}{2} \rho S_w C_L v ^2$ |
ID:(4417, 0)
Equilibrium lifting coefficient
Equation
The condition for achieving flight is met when the lift force ($F_L$) equals the weight of the aircraft or bird, which is calculated from the mass of Object ($m$) and the gravitational Acceleration ($g$). This is achieved with sufficient values of speed with respect to the medium ($v$), the surface that generates lift ($S_w$), and the coefficient of lift ($C_L$), where the latter coefficient is the adjustable factor. In the case of aircraft, pilots can modify the value of the coefficient of lift ($C_L$) using flaps, whose value must satisfy:
| $ C_L =\displaystyle\frac{2 m g }{ \rho S_w }\displaystyle\frac{1}{ v ^2}$ |
The lift force ($F_L$) along with the density ($\rho$), the surface that generates lift ($S_w$), the coefficient of lift ($C_L$), and the speed with respect to the medium ($v$) is represented as
| $ F_L =\displaystyle\frac{1}{2} \rho S_w C_L v ^2$ |
which, along with the mass of Object ($m$) and the gravitational Acceleration ($g$), must be equal to:
| $ F_g = m g $ |
that is:
$\displaystyle\frac{1}{2}\rho S_wC_Lv^2=mg$
resulting in:
| $ C_L =\displaystyle\frac{2 m g }{ \rho S_w }\displaystyle\frac{1}{ v ^2}$ |
Flaps are adjusted by changing the angle that the wing makes with the direction of flight, known as the angle of attack.
ID:(4442, 0)
Lift constant
Equation
From measurements, it is concluded that the lift coefficient $C_L$ is proportional to the angle of attack $\alpha$:
| $ C_L = c \alpha $ |
After a certain angle, the curve decreases until it reaches zero. This is because beyond that critical angle, the vortices fully cover the upper surface of the wing, leading to a loss of lift. This phenomenon is known as \"stall\".
ID:(4441, 0)
Attack angle
Equation
Since the lift coefficient $C_L$ is proportional to the angle of attack $\alpha$, we can calculate the necessary angle to achieve sufficient lift for a given velocity $v$:
| $ \alpha =\displaystyle\frac{2 m g }{ c \rho S_w }\displaystyle\frac{1}{ v ^2}$ |
The coefficient of lift ($C_L$) is calculated with the mass of Object ($m$), the gravitational Acceleration ($g$), the surface that generates lift ($S_w$), the density ($\rho$), and the speed with respect to the medium ($v$) as follows:
| $ C_L =\displaystyle\frac{2 m g }{ \rho S_w }\displaystyle\frac{1}{ v ^2}$ |
Therefore, with the proportionality constant coefficient sustainability ($c$) and the angle of attack of a wing ($\alpha$),
| $ C_L = c \alpha $ |
we have
| $ \alpha =\displaystyle\frac{2 m g }{ c \rho S_w }\displaystyle\frac{1}{ v ^2}$ |
where $m$ is the mass, $g$ is the gravitational acceleration, $\rho$ is the density of the medium, $S_w$ is the wing area, and $c$ is the proportionality constant between the lift coefficient and the angle of attack.
ID:(4443, 0)
Speed $V2$
Equation
Takeoff occurs when the speed with respect to the medium ($v$) reaches a sufficient threshold for the angle of attack of a wing ($\alpha$), influenced by the flaps and the rotation of the aircraft, to meet the following condition:
| $ \alpha =\displaystyle\frac{2 m g }{ c \rho S_w }\displaystyle\frac{1}{ v ^2}$ |
considering the mass of Object ($m$), the density ($\rho$), the gravitational Acceleration ($g$), the total object profile ($S_p$), and the proportionality constant coefficient sustainability ($c$).
In this scenario, the angle of attack of a wing ($\alpha$) equals the angle required for lift ($\alpha_s$), and the speed with respect to the medium ($v$) equals the critical speed $V2$ ($V2$):
| $ V2 = \sqrt{\displaystyle\frac{2 m g }{ c \rho S_w \alpha_s }}$ |
ID:(14477, 0)
Rotation speed $Vr$
Equation
The rotation speed $Vr$ ($Vr$) is achieved when the aircraft can take off by adjusting its ascent angle. In other words, it corresponds to the scenario of the critical speed $V2$ ($V2$) with the mass of Object ($m$), the gravitational Acceleration ($g$), the proportionality constant coefficient sustainability ($c$), the gravitational Acceleration ($g$), the total object profile ($S_p$), and the angle required for lift ($\alpha_s$):
| $ V2 = \sqrt{\displaystyle\frac{2 m g }{ c \rho S_w \alpha_s }}$ |
when the aircraft has not yet rotated, and the angle required for lift ($\alpha_s$) equals the angle of attack of a wing ($\alpha$), which does not account for the aircraft's rotation. In essence:
| $ Vr = \sqrt{\displaystyle\frac{2 m g }{ c \rho S_w \alpha }}$ |
ID:(14474, 0)
Acceleration on takeoff
Equation
Essentially, aircraft use propulsion systems to achieve the necessary acceleration. This the propulsion force ($F_p$) is countered by the resistance force ($F_W$), which, along with the density ($\rho$), the total object profile ($S_p$), the coefficient of resistance ($C_W$), and the speed with respect to the medium ($v$), follows the equation
| $ F_W =\displaystyle\frac{1}{2} \rho S_p C_W v ^2$ |
.
Therefore, the total force is equal to the product of the mass of Object ($m$) and the acceleration ($a$), which can be expressed as the variation of the speed with respect to the medium ($v$) in terms of time ($t$), as indicated by the label
| $ m \displaystyle\frac{dv}{dt} = F_p - \displaystyle\frac{1}{2} \rho S_p C_W v ^2$ |
The total force is equal to the propulsion force ($F_p$), countered by the resistance force ($F_W$) along with the density ($\rho$), the coefficient of resistance ($C_W$), the total object profile ($S_p$), and the speed with respect to the medium ($v$) according to the equation
| $ F_W =\displaystyle\frac{1}{2} \rho S_p C_W v ^2$ |
,
resulting in the following equation:
$F = F_p - \displaystyle\frac{1}{2} \rho S_w C_L v ^2$
Since the total force equals the mass of Object ($m$) multiplied by the acceleration ($a$), and the latter represents the change in the speed with respect to the medium ($v$) with respect to time ($t$), we obtain the following differential equation:
$F = m a = m \displaystyle\frac{dv}{dt}$
This leads to the differential equation:
| $ m \displaystyle\frac{dv}{dt} = F_p - \displaystyle\frac{1}{2} \rho S_p C_W v ^2$ |
.
.
ID:(14505, 0)
Initial acceleration
Equation
At the beginning of takeoff, aerodynamic resistance, which depends on velocity, is minimal. Therefore, the maximum acceleration ($a_p$) is determined solely by the propulsion force ($F_p$) and the mass of Object ($m$):
| $ a_p = \displaystyle\frac{ F_p }{ m }$ |
As aerodynamic resistance starts to reduce the propulsion force, this initial acceleration will be the maximum possible.
ID:(14506, 0)
Maximum speed
Equation
The propulsion force ($F_p$) counteracts the resistance force ($F_W$) by generating velocity, which in turn increases the same resistance force, as described in the total object profile ($S_p$), the coefficient of resistance ($C_W$), the density ($\rho$), and the speed with respect to the medium ($v$) in
| $ F_W =\displaystyle\frac{1}{2} \rho S_p C_W v ^2$ |
This process continues to increase the velocity until the point where the propulsion force equals the resistance force, representing the maximum achievable speed.
By equating the propulsion force with the resistance force and solving for velocity, we obtain the maximum speed ($v_p$):
| $ v_p = \sqrt{\displaystyle\frac{2 F_p }{ \rho S_p C_W } }$ |
If we equate the propulsion force ($F_p$) with the resistance force ($F_W$) with the total object profile ($S_p$), the coefficient of resistance ($C_W$), the density ($\rho$), and the speed with respect to the medium ($v$) in
| $ F_W =\displaystyle\frac{1}{2} \rho S_p C_W v ^2$ |
we obtain, for a the maximum speed ($v_p$),
$F_p = \displaystyle\frac{1}{2} \rho S_w C_L v_p ^2$
which, when solved for the maximum velocity, results in
| $ v_p = \sqrt{\displaystyle\frac{2 F_p }{ \rho S_p C_W } }$ |
As aerodynamic resistance starts to reduce the propulsion force, this initial acceleration will be the maximum possible.
ID:(14507, 0)
Takeoff speed equation
Equation
The equation for an airplane taking off with speed with respect to the medium ($v$) can be rewritten as follows when it takes off with the density ($\rho$), the total object profile ($S_p$), the coefficient of resistance ($C_W$), the mass of Object ($m$), the time ($t$), and the propulsion force ($F_p$):
| $ m \displaystyle\frac{dv}{dt} = F_p - \displaystyle\frac{1}{2} \rho S_p C_W v ^2$ |
It can be rewritten with the maximum acceleration ($a_p$) and the maximum speed ($v_p$) as:
| $\displaystyle\frac{dv}{dt}=a_p\left[1- \left(\displaystyle\frac{v}{v_p}\right)^2\right]$ |
The equation for an airplane taking off with speed with respect to the medium ($v$) can be rewritten as follows when it takes off with the density ($\rho$), the total object profile ($S_p$), the coefficient of resistance ($C_W$), the mass of Object ($m$), the time ($t$), and the propulsion force ($F_p$):
| $ m \displaystyle\frac{dv}{dt} = F_p - \displaystyle\frac{1}{2} \rho S_p C_W v ^2$ |
This can be expressed as:
| $ a_p = \displaystyle\frac{ F_p }{ m }$ |
and the maximum speed ($v_p$)
| $ v_p = \sqrt{\displaystyle\frac{2 F_p }{ \rho S_p C_W } }$ |
as follows:
| $\displaystyle\frac{dv}{dt}=a_p\left[1- \left(\displaystyle\frac{v}{v_p}\right)^2\right]$ |
ID:(15158, 0)
Takeoff speed
Equation
The speed with respect to the medium ($v$) for an airplane taking off satisfies the equation with the maximum acceleration ($a_p$), the maximum speed ($v_p$), and the time ($t$):
| $\displaystyle\frac{dv}{dt}=a_p\left[1- \left(\displaystyle\frac{v}{v_p}\right)^2\right]$ |
When integrated in the limit speed with respect to the medium ($v$) much smaller than maximum speed ($v_p$), we obtain:
| $ v = \sqrt{ 2 a_p v_p t }$ |
The speed with respect to the medium ($v$) for an airplane taking off satisfies the equation with the maximum acceleration ($a_p$), the maximum speed ($v_p$), and the time ($t$):
| $\displaystyle\frac{dv}{dt}=a_p\left[1- \left(\displaystyle\frac{v}{v_p}\right)^2\right]$ |
Upon integration, we obtain the following expression:
$\log(v_p + v) + \log(v_p - v) = \displaystyle\frac{2 a_p}{v_p} t$
If the speed with respect to the medium ($v$) is much smaller than the maximum speed ($v_p$), the logarithms can be expanded into a Taylor series, resulting in a first-order approximation:
| $ v = \sqrt{ 2 a_p v_p t }$ |
Typically, the takeoff speed of an aircraft is significantly lower than the maximum speed the maximum speed ($v_p$). Therefore, the equation can be solved analytically, as explained in the development.
ID:(14508, 0)
Path taken when taking off
Equation
Since the takeoff velocity $v$ as a function of time $t$ is described by
| $ v = \sqrt{ 2 a_p v_p t }$ |
we can calculate the distance traveled along the runway by integrating this equation with respect to time:
| $ s = \sqrt{\displaystyle\frac{ 2^3 a_p v_p t ^3}{3^2}}$ |
Since the velocity as a function of time is given by
| $ v = \sqrt{ 2 a_p v_p t }$ |
we can express velocity as the rate of change of distance with respect to time:
$\displaystyle\frac{ds}{dt} = \sqrt{2 a_p v_p t }$
This equation can be integrated, yielding the relationship between the distance traveled and time:
| $ s = \sqrt{\displaystyle\frac{ 2^3 a_p v_p t ^3}{3^2}}$ |
On the other hand, by considering the required takeoff velocity, we can determine the time needed to achieve it, and using the distance traveled, we can calculate the runway length required for takeoff.
ID:(14509, 0)
0
Video
Video: Take off and land