User:
Hooke's Law
Equation
The relationship between the elastic Force ($F_k$) and elongation the elongation ($u$) is written and referred to as Hooke's Law. The constant the hooke Constant ($k$) is called the spring constant:
 $ F_k = k u $ |
ID:(3207, 0)
Two springs in series
Concept
If you want to model how a solid deforms under the influence of a force, you can first consider the behavior of a subunit, such as two springs connected one behind the other, as shown in the image:
This type of arrangement of the springs is called in series. It is characterized by the fact that the force the average force ($\bar{F}$) is the same in both springs, and they deform according to the hooke Constant ($k$). Therefore, the equivalent spring constant the elongation ($u$) is calculated as the sum of the spring elongation 1 ($u_1$) and the spring elongation 2 ($u_2$), which, in turn, by Hooke's law:
| $ F_k = k u $ |
is equal to the average force ($\bar{F}$) divided by the constants the spring Hook constant 1 ($k_1$) and the spring Hook constant 2 ($k_2$), respectively:
$u = u_1 + u_2 = \displaystyle\frac{F}{k_1} + \displaystyle\frac{F}{k_2} = \left(\displaystyle\frac{1}{k_1} + \displaystyle\frac{1}{k_2}\right)F$
Therefore, the system of two springs can be treated as a single spring whose equivalent spring constant the total Hook constant of springs in series ($k_s$) is calculated as follows:
| $\displaystyle\frac{1}{ k_s }=\displaystyle\frac{1}{ k_1 }+\displaystyle\frac{1}{ k_2 }$ |
ID:(1910, 0)
Sum of Springs in Series
Equation
In the case of two resistors with values the spring Hook constant 1 ($k_1$) and the spring Hook constant 2 ($k_2$), when they are connected in parallel, they act as if there were an equivalent resistance the total Hook constant of springs in series ($k_s$) given by the following equation:
| $\displaystyle\frac{1}{ k_s }=\displaystyle\frac{1}{ k_1 }+\displaystyle\frac{1}{ k_2 }$ |
This concept can be generalized for the spring Hook constant i ($k_i$) as:
| $\displaystyle\frac{1}{ k_s }=\displaystyle\sum_i\displaystyle\frac{1}{ k_i }$ |
When we apply forces the average force ($\bar{F}$) at the ends of the springs, the springs will elongate (or compress) by the spring elongation i ($u_i$) and the spring Hook constant i ($k_i$) respectively. If the point of contact between both springs is at rest, the sum of the forces acting on it must add up to zero, meaning they must be equal to the average force ($\bar{F}$). Therefore, for each spring $i$, it must satisfy
$F = k_iu_i$
The total elongation will be equal to the sum of the individual elongations:
$u = \displaystyle\sum_iu_i$
And using Hooke's law, this can be expressed as:
$u = \displaystyle\sum_i\frac{F}{k_i}$
If we introduce a total constant for the case of a series connection the total Hook constant of springs in series ($k_s$), such that
$F = k_su$
Then we have:
| $\displaystyle\frac{1}{ k_s }=\displaystyle\sum_i\displaystyle\frac{1}{ k_i }$ |
ID:(3208, 0)
Springs in Series (2)
Equation
If we have two resistors with values the spring Hook constant 1 ($k_1$) and the spring Hook constant 2 ($k_2$) connected in series, the extensions add up, causing each individual resistor to act based on its inverse. In this way, the inverse of the total Hook constant of springs in series ($k_s$) is equal to the sum of the inverses of the individual constants the spring Hook constant i ($k_i$):
| $\displaystyle\frac{1}{ k_s }=\displaystyle\frac{1}{ k_1 }+\displaystyle\frac{1}{ k_2 }$ |
Since each spring is exposed to the same applied force the average force ($\bar{F}$), the springs with constants the spring Hook constant 1 ($k_1$) and the spring Hook constant 2 ($k_2$) will deform by magnitudes the spring elongation 1 ($u_1$) and the spring elongation 2 ($u_2$) respectively, according to the following equations:
$F = k_1u_1$
$F = k_2u_2$
The total elongation will be the sum of both elongations:
$u = u_1 + u_2 = \displaystyle\frac{F}{k_1} + \displaystyle\frac{F}{k_2} = \left(\displaystyle\frac{1}{k_1} + \displaystyle\frac{1}{k_2}\right)F$
Therefore, the system behaves as if it had a spring constant equal to:
| $\displaystyle\frac{1}{ k_s }=\displaystyle\frac{1}{ k_1 }+\displaystyle\frac{1}{ k_2 }$ |
ID:(3753, 0)
Springs in Series (3)
Equation
$\displaystyle\frac{1}{k_s}=\displaystyle\frac{1}{k_1}+\displaystyle\frac{1}{k_2}+\displaystyle\frac{1}{k_3}$
ID:(3754, 0)
Two Springs in Parallel
Concept
If one wishes to model how a solid deforms under the influence of a force, the behavior of a subunit can first be considered, such as two springs connected side by side, as shown in the image:
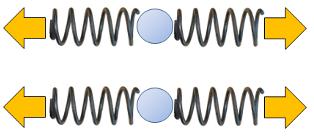
This type of arrangement of the springs is called in parallel. It is characterized because the elongation ($u$) in both springs is the same and each spring contributes the average force ($\bar{F}$) according to the spring Hook constant 1 ($k_1$) and the spring Hook constant 2 ($k_2$) as per Hooke's law:
| $ F_k = k u $ |
Thus, it follows that:
$F = F_1 + F_2 = k_1 u + k_2 u = (k_1 + k_2)u$
Therefore, the system of two springs can be treated as a single spring whose equivalent spring constant the total Hook constant of springs in parallel ($k_p$) is calculated as follows:
| $ k_p = k_1 + k_2 $ |
ID:(1692, 0)
Sum of multiple springs in parallel
Concept
In the case of two springs with constants the spring Hook constant 1 ($k_1$) and the spring Hook constant 2 ($k_2$) that can be modeled by a single spring with a constant the total Hook constant of springs in parallel ($k_p$) calculated using the following equation:
| $ k_p = k_1 + k_2 $ |
For the more general case of springs with constants spring Hook constant i ($k_i$), the equation can be generalized as follows:
| $ k_p =\displaystyle\sum_i k_i$ |
This allows us to model a macro structure as follows:
ID:(1684, 0)
Sum of springs in parallel
Equation
For the case of two resistors with values the spring Hook constant 1 ($k_1$) and the spring Hook constant 2 ($k_2$), when they are connected in parallel, they act as if there were an equivalent resistance the total Hook constant of springs in parallel ($k_p$) given by the following equation:
| $ k_p = k_1 + k_2 $ |
This concept can be generalized for the spring Hook constant i ($k_i$) as:
| $ k_p =\displaystyle\sum_i k_i$ |
Since each spring can have a different spring constant, represented by the spring Hook constant i ($k_i$), the force contributed by each spring also varies. According to Hooke's law, the forces $F_i$ can be expressed as:
$F_i = k_i u$
As the total force $F$ corresponds to the sum of individual forces, we have:
$F =\displaystyle\sum_i F_i = \displaystyle\sum_i k_i u$
Therefore, a total spring constant can be defined as:
| $ k_p =\displaystyle\sum_i k_i$ |
ID:(3756, 0)
Springs in Parallel (2)
Equation
If you have two resistors with values the spring Hook constant 1 ($k_1$) and the spring Hook constant 2 ($k_2$) connected in parallel, their effects add up, acting as if there were an equivalent resistance the total Hook constant of springs in parallel ($k_p$) equal to the sum of the individual constants:
| $ k_p = k_1 + k_2 $ |
Since each spring is subjected to the same the elongation ($u$), the forces will be different if the spring constants are different. Therefore, if the spring Hook constant 1 ($k_1$) and the spring Hook constant 2 ($k_2$) are the spring constants, the forces will be:
$F_1 = k_1 u$
$F_2 = k_2 u$
As a result, the total force will be:
$F = F_1 + F_2 = k_1u + k_2u = (k_1 + k_2)u$
Thus, the system behaves as if it had a spring constant equal to:
| $ k_p = k_1 + k_2 $ |
ID:(3757, 0)
Number of springs connected in parallel
Equation
To calculate the macroscopic equivalent of the microscopic spring constant, one must sum all the microsprings both in parallel and in series. To do this, it is necessary to know the number of springs connected in parallel.
The number of springs connected in parallel can be determined with body Section ($S$) and the microscopic section of spring ($s$). The number of springs in parallel ($N_p$) is calculated by dividing body Section ($S$) by the microscopic section of spring ($s$):
| $ N_p =\displaystyle\frac{ S }{ s }$ |
ID:(3760, 0)
Number of Springs connected in Series
Equation
To calculate the macroscopic equivalent constant of the microscopic spring constant, all microsprings must be added both in parallel and in series. To do this, it is necessary to know, in particular, the number of springs connected in series.
If we want to estimate the number of springs in series ($N_s$), it is sufficient to know the body length ($L$) and microscopic Length of Spring ($l$). The number of springs in series ($N_s$) is calculated by dividing the body length ($L$) by microscopic Length of Spring ($l$):
| $ N_s =\displaystyle\frac{ L }{ l }$ |
ID:(3761, 0)
Macroscopic element model
Equation
For a bar with ($$) and body Section ($S$), you can calculate the number of springs in parallel ($N_p$) and the number of springs in series ($N_s$) using microscopic Length of Spring ($l$) and the microscopic section of spring ($s$). With these values, you can calculate the spring constant for an entire section by multiplying by the number of springs in parallel ($N_p$) using the microscopic Hook constant ($k_m$). This way, you can calculate the hooke Constant ($k$) by dividing the obtained value by the number of springs in series ($N_s$):
$k=\displaystyle\frac{k_p}{N_s}=\displaystyle\frac{N_p}{N_s}k_m$
If you introduce the expressions for the number of elements with microscopic Length of Spring ($l$) and the microscopic section of spring ($s$), you get the following expression:
| $ k =\displaystyle\frac{ S }{ L }\displaystyle\frac{ l }{ s } k_m $ |
As the the total Hook constant of springs in parallel ($k_p$) of the spring Hook constant i ($k_i$) is
| $ k_p =\displaystyle\sum_i k_i$ |
it follows that in the case of the microscopic Hook constant ($k_m$) being equal to
$k_p = N_p k_m$
the total Hook constant of springs in parallel ($k_p$) corresponds, in this case, to the Hook's constant of a monoatomic thickness section. To obtain the constant for the entire body, one must sum all the sections in series, and for this, we work with the relationship for the sum of the total Hook constant of springs in series ($k_s$), given by
| $\displaystyle\frac{1}{ k_s }=\displaystyle\sum_i\displaystyle\frac{1}{ k_i }$ |
With the number of sections being equal to the number of springs in series ($N_s$), and if we assume that they are all equal, we obtain
$\displaystyle\frac{1}{k} = N_s\displaystyle\frac{1}{N_p k_m}$
meaning
$k = \displaystyle\frac{N_p}{N_s}k_m$
Finally, with the relationships for the body length ($L$) and microscopic Length of Spring ($l$)
| $ N_s =\displaystyle\frac{ L }{ l }$ |
and with body Section ($S$) and the microscopic section of spring ($s$)
| $ N_p =\displaystyle\frac{ S }{ s }$ |
we ultimately obtain
| $ k =\displaystyle\frac{ S }{ L }\displaystyle\frac{ l }{ s } k_m $ |
ID:(3205, 0)
Modulus of elasticity
Equation
The expression for the hooke Constant ($k$) given by
| $ k =\displaystyle\frac{ S }{ L }\displaystyle\frac{ l }{ s } k_m $ |
has two macroscopic parameters, which are the body length ($L$) and body Section ($S$). The remaining the microscopic Hook constant ($k_m$), microscopic Length of Spring ($l$), and the microscopic section of spring ($s$) are microscopic and thus depend on the material being described. Therefore, it makes sense to define these factors as the modulus of Elasticity ($E$), so that:
| $ E =\displaystyle\frac{ l }{ s } k_m $ |
ID:(3204, 0)
Hooke force of an object
Equation
As Hooke's Law relates the elastic Force ($F_k$) through the hooke Constant ($k$) and the elongation ($u$) in the following manner:
| $ F_k = k u $ |
you can replace the hooke Constant ($k$) with the microscopic expression and using the definition of the modulus of Elasticity ($E$), you obtain with the body length ($L$) and body Section ($S$) that:
| $ F_k =\displaystyle\frac{ E S }{ L } u $ |
With Hooke's Law for the elastic Force ($F_k$), the hooke Constant ($k$), and the elongation ($u$) as follows:
| $ F_k = k u $ |
and the expression for the hooke Constant ($k$) in terms of the body length ($L$), body Section ($S$), microscopic Length of Spring ($l$), the microscopic section of spring ($s$), and the microscopic Hook constant ($k_m$):
| $ k =\displaystyle\frac{ S }{ L }\displaystyle\frac{ l }{ s } k_m $ |
combined with the expression for the modulus of Elasticity ($E$):
| $ E =\displaystyle\frac{ l }{ s } k_m $ |
the result is:
| $ F_k =\displaystyle\frac{ E S }{ L } u $ |
ID:(3209, 0)
Deformation
Equation
The elastic Force ($F_k$) is a function of the modulus of Elasticity ($E$), body Section ($S$), the elongation ($u$), and the body length ($L$).
| $ F_k =\displaystyle\frac{ E S }{ L } u $ |
In this case, the ratio between the elongation ($u$) and the body length ($L$) is represented by the deformation ($\epsilon$), which can be defined as follows:
| $ \epsilon =\displaystyle\frac{ u }{ L }$ |
ID:(3762, 0)
Stress
Equation
The elastic Force ($F_k$) is a function that depends on the modulus of Elasticity ($E$), body Section ($S$), the elongation ($u$), and the body length ($L$).
| $ F_k =\displaystyle\frac{ E S }{ L } u $ |
Similarly, just as the deformation ($\epsilon$) is introduced to avoid using the dimension the body length ($L$), we can construct a factor that expresses the elastic Force ($F_k$) in terms of body Section ($S$) as the strain ($\sigma$).
| $ \sigma =\displaystyle\frac{ F }{ S }$ |
ID:(3210, 0)
Hooke's law continues by direction
Equation
The Hooke's Law for tensión ($\sigma$), modulo de elasticidad ($E$), and deformación ($\epsilon$) is expressed as:
| $ \sigma = E \epsilon $ |
This law can be generalized for the stress on axis $i$ ($\sigma_i$) and the deformation in the coordinate $i$ ($\epsilon_i$) as:
| $ \sigma_i = E \epsilon_i $ |
ID:(3764, 0)
Deformation as continuum
Equation
In general, the deformation ($\epsilon$) is defined as the variation of the elongation ($u$) in proportion to the body length ($L$):
| $ \epsilon =\displaystyle\frac{ u }{ L }$ |
This concept can be generalized in the microscopic limit, where the deformation in the coordinate $i$ ($\epsilon_i$) is introduced as the variation of displacement in i ($\partial u_i$) over the length of an element in i ($\partial x_i$) in the direction $i$, and it would be expressed as:
| $ \epsilon_i =\displaystyle\frac{\partial u_i }{\partial x_i }$ |
The reason for using a different symbol to denote the differential
$d \rightarrow \partial$
is that there are several differentials that affect different variables in the model. The use of the symbol $\partial$ indicates that one should perform one variation at a time, meaning that when considering one variable, the remaining variables are assumed to have their initial values.
ID:(3763, 0)
Hooke's Law for the Case Shear
Equation
In the case of shear, the deformation is not associated with expanding or compressing, but with laterally offsetting the faces of a cube. The shear is therefore described by the angle
| $ \tau = G \gamma $ |
where
ID:(3771, 0)
Poisson's ratio
Equation
Lateral deformation is directly proportional to the deformation it causes. The proportionality coefficient is denoted as the poisson coefficient ($\nu$) [1] and typically falls within the range of 0.15 to 0.4.
If the original deformation is the deformation ($\epsilon$) and the generated one is the deformation in the direction perpendicular to the force ($\epsilon_{\perp}$), the following relationship is established:
In the linear approximation, the Poisson's coefficient represents the relationship between lateral and longitudinal deformations.
| $ \epsilon_{\perp} =- \nu \epsilon $ |
where the sign indicates that the deformation is in the opposite direction to the cause.
[1] This concept was introduced by Siméon Denis Poisson in a statistical analysis work, in which he mentioned, among other unrelated topics to mechanics, what was later referred to as the Poisson's coefficient in an elasticity example. The work is titled "Recherches sur la Probabilité des Jugements en Matière Criminelle et en Matière Civile" (Research on the Probability of Judgments in Criminal and Civil Matters), authored by Siméon Denis Poisson (1837).
ID:(3765, 0)
Moment of Surface Inertia
Equation
| $ I_s =\displaystyle\frac{ \pi }{2}( R_2 ^4- R_1 ^4)$ |
None
ID:(3774, 0)